102. 二叉树的层序遍历

🟠   🔖  树 广度优先搜索 二叉树  🔗 力扣 LeetCode

题目

Given the root of a binary tree, return the level order traversal of its nodes ' values. (i.e., from left to right, level by level).

Example 1:

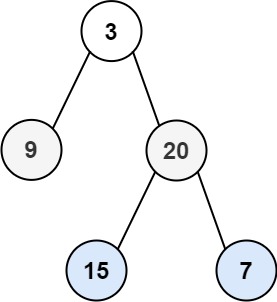
Input: root = [3,9,20,null,null,15,7]

Output: [[3],[9,20],[15,7]]

Example 2:

Input: root = [1]

Output: [[1]]

Example 3:

Input: root = []

Output: []

Constraints:

• The number of nodes in the tree is in the range [0, 2000].
• -1000 <= Node.val <= 1000

题目大意

给你二叉树的根节点 root ，返回它节点值的 层序 遍历。（即逐层地，从左到右访问所有节点）。

解题思路

思路一：广度优先遍历(BFS)

• 使用队列实现

1. 首先将根节点放入队列中；
2. 更新队列的长度 len ，遍历队列的前 len 个节点；
3. 如果该节点存在直接子节点，将直接子节点加入队列中，并将节点的值存入一个临时数组中；
4. 将队列的前 len 个节点出队，此时队列中都是下一层的子节点，将临时数组加入返回值中；
5. 重复步骤 2、3、4，直至队列为空；

---

思路二：深度优先遍历(DFS)

1. 维护一个递归函数，参数为节点和该节点的深度
2. 先将根节点与深度 0 传入递归函数
3. 将节点放入 index 与深度对应的数组内
4. 将节点的左子节点和右子节点分别传入递归函数，深度 +1
5. 重复步骤 3、4，直至子节点为空

代码

广度优先遍历(BFS)

// 思路一：广度优先遍历(BFS)
/**
 * @param {TreeNode} root
 * @return {number[][]}
 */
var levelOrder = function (root) {
	let res = [];
	if (root == null) return res;
	let queue = [root];

	while (queue.length) {
		let len = queue.length;
		let temp = [];
		for (let i = 0; i < len; i++) {
			if (queue[i].left) queue.push(queue[i].left);
			if (queue[i].right) queue.push(queue[i].right);
			temp.push(queue[i].val);
		}
		queue = queue.slice(len);
		res.push(temp);
	}
	return res;
};

深度优先遍历(DFS)

// 思路二：深度优先遍历(DFS)
/**
 * @param {TreeNode} root
 * @return {number[][]}
 */
var levelOrder = function (root) {
	let res = [];
	const traverse = (node, deep) => {
		if (node == null) return;
		if (res.length == deep) {
			res[deep] = [node.val];
		} else {
			res[deep].push(node.val);
		}
		traverse(node.left, deep + 1);
		traverse(node.right, deep + 1);
	};
	traverse(root, 0);
	return res;
};

相关题目

题号	标题	题解	标签	难度	力扣
103	二叉树的锯齿形层序遍历	[✓]	树 广度优先搜索 二叉树	🟠	🀄️ 🔗
107	二叉树的层序遍历 II	[✓]	树 广度优先搜索 二叉树	🟠	🀄️ 🔗
111	二叉树的最小深度	[✓]	树 深度优先搜索 广度优先搜索 1+	🟢	🀄️ 🔗
314	二叉树的垂直遍历 🔒		树 深度优先搜索 广度优先搜索 3+	🟠	🀄️ 🔗
429	N 叉树的层序遍历		树 广度优先搜索	🟠	🀄️ 🔗
637	二叉树的层平均值	[✓]	树 深度优先搜索 广度优先搜索 1+	🟢	🀄️ 🔗
993	二叉树的堂兄弟节点		树 深度优先搜索 广度优先搜索 1+	🟢	🀄️ 🔗
2471	逐层排序二叉树所需的最少操作数目		树 广度优先搜索 二叉树	🟠	🀄️ 🔗
2493	将节点分成尽可能多的组		广度优先搜索 并查集 图	🔴	🀄️ 🔗