235. 二叉搜索树的最近公共祖先

🟠   🔖  树 深度优先搜索 二叉搜索树 二叉树  🔗 力扣 LeetCode

题目

Given a binary search tree (BST), find the lowest common ancestor (LCA) node of two given nodes in the BST.

According to the definition of LCA on Wikipedia: "The lowest common ancestor is defined between two nodes p and q as the lowest node in T that has both p and q as descendants (where we allow a node to be a descendant of itself )."

Example 1:

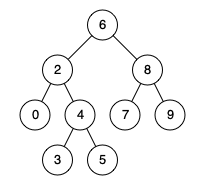
Input: root = [6,2,8,0,4,7,9,null,null,3,5], p = 2, q = 8

Output: 6

Explanation: The LCA of nodes 2 and 8 is 6.

Example 2:

Input: root = [6,2,8,0,4,7,9,null,null,3,5], p = 2, q = 4

Output: 2

Explanation: The LCA of nodes 2 and 4 is 2, since a node can be a descendant of itself according to the LCA definition.

Example 3:

Input: root = [2,1], p = 2, q = 1

Output: 2

Constraints:

• The number of nodes in the tree is in the range [2, 10^5].
• -10^9 <= Node.val <= 10^9
• All Node.val are unique.
• p != q
• p and q will exist in the BST.

题目大意

给定一个二叉搜索树, 找到该树中两个指定节点的最近公共祖先。

维基百科中最近公共祖先的定义为：“对于有根树 T 的两个结点 p、q，最近公共祖先表示为一个结点 x，满足 x 是 p、q 的祖先且 x 的深度尽可能大（一个节点也可以是它自己的祖先）。”

解题思路

思路一：递归比数值

由于 BST 的性质，可以通过比较节点的值来确定最近的公共祖先。

1. 如果节点的值小于 p 和 q 的值，说明 p 和 q 都在节点的右子树中，递归搜索右子树。
2. 如果节点的值大于 p 和 q 的值，说明 p 和 q 都在节点的左子树中，递归搜索左子树。
3. 如果节点的值在 p 和 q 的值之间，说明当前节点即为最近的公共祖先。

复杂度分析

• 时间复杂度：O(h)，其中 h 是树的高度，在二叉搜索树中，查找最近公共祖先的时间复杂度为 O(h)。
  • 对于平衡的二叉搜索树，h = log n，其中 n 是树中节点的总数，因此时间复杂度在平衡情况下为 O(log n)。
  • 在最坏情况下（例如树呈线性结构），时间复杂度为 O(n)。
• 空间复杂度：O(h)，其中 h 是树的高度，空间复杂度主要由递归调用栈的深度决定。
  • 对于平衡的二叉搜索树，空间复杂度为 O(log n)，其中 n 是树中节点的总数。
  • 在最坏情况下（例如，树是完全不平衡的），递归的深度可以达到 O(n)。

---

思路二：递归查找

和 第 236 题 一样，使用递归查找。

从根节点开始，递归地向左子树和右子树搜索。递归的终止条件有几种情况：

1. 如果当前节点为 null，表示遍历到空节点，直接返回 null。
2. 如果当前节点等于 p 或 q，表示找到了其中一个节点，直接返回当前节点。

递归步骤如下：

1. 递归地在左子树中寻找 p 和 q 的最低共同祖先，结果存储在变量 left 中。
2. 递归地在右子树中寻找 p 和 q 的最低共同祖先，结果存储在变量 right 中。

然后，根据 left 和 right 的情况，可以得出以下结论：

• 如果 left 和 right 都不为 null，说明 p 和 q 分别位于当前节点的左右子树，因此当前节点就是它们的最低共同祖先，直接返回当前节点。
• 如果只有 left 不为 null，说明 p 和 q 都在左子树，最低共同祖先在左子树中，返回 left。
• 如果只有 right 不为 null，说明 p 和 q 都在右子树，最低共同祖先在右子树中，返回 right。

复杂度分析

• 时间复杂度：O(n)，其中 n 是树中节点的总数，因为在最坏的情况下，需要检查每个节点来找到最近公共祖先。
• 空间复杂度：O(h)，其中 h 是树的高度，空间复杂度主要由递归调用栈的深度决定。
  • 在平衡树中，递归深度为 O(h)，其中 h 是树的高度。
  • 在最坏情况下（例如，树是完全不平衡的），递归的深度可以达到 O(n)，其中 n 是树中节点的总数。

代码

递归比数值

/**
 * @param {TreeNode} root
 * @param {TreeNode} p
 * @param {TreeNode} q
 * @return {TreeNode}
 */
var lowestCommonAncestor = function (root, p, q) {
	if (!root) return null;
	if (root.val < p.val && root.val < q.val) {
		return lowestCommonAncestor(root.right, p, q);
	} else if (root.val > p.val && root.val > q.val) {
		return lowestCommonAncestor(root.left, p, q);
	}
	return root;
};

递归查找

/**
 * @param {TreeNode} root
 * @param {TreeNode} p
 * @param {TreeNode} q
 * @return {TreeNode}
 */
var lowestCommonAncestor = function (root, p, q) {
	if (!root || p == root || q == root) return root;
	let left = lowestCommonAncestor(root.left, p, q);
	let right = lowestCommonAncestor(root.right, p, q);
	if (left && right) return root;
	return left ? left : right;
};

相关题目

题号	标题	题解	标签	难度	力扣
236	二叉树的最近公共祖先	[✓]	树 深度优先搜索 二叉树	🟠	🀄️ 🔗
1257	最小公共区域 🔒		树 深度优先搜索 广度优先搜索 3+	🟠	🀄️ 🔗
1644	二叉树的最近公共祖先 II 🔒		树 深度优先搜索 二叉树	🟠	🀄️ 🔗
1650	二叉树的最近公共祖先 III 🔒		树 哈希表 双指针 1+	🟠	🀄️ 🔗
1676	二叉树的最近公共祖先 IV 🔒		树 深度优先搜索 哈希表 1+	🟠	🀄️ 🔗