766. 托普利茨矩阵
766. 托普利茨矩阵
题目
Given an m x n matrix, return true if the matrix is Toeplitz. Otherwise, return false.
A matrix is Toeplitz if every diagonal from top-left to bottom-right has the same elements.
Example 1:
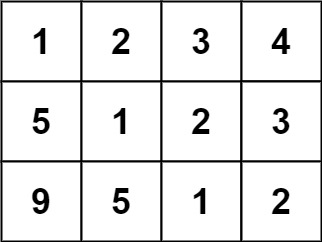
Input: matrix = [[1,2,3,4],[5,1,2,3],[9,5,1,2]]
Output: true
Explanation:
In the above grid, the diagonals are:
"[9]", "[5, 5]", "[1, 1, 1]", "[2, 2, 2]", "[3, 3]", "[4]".
In each diagonal all elements are the same, so the answer is True.
Example 2:
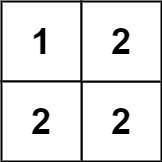
Input: matrix = [[1,2],[2,2]]
Output: false
Explanation:
The diagonal "[1, 2]" has different elements.
Constraints:
m == matrix.lengthn == matrix[i].length1 <= m, n <= 200 <= matrix[i][j] <= 99
Follow up:
- What if the
matrixis stored on disk, and the memory is limited such that you can only load at most one row of the matrix into the memory at once? - What if the
matrixis so large that you can only load up a partial row into the memory at once?
题目大意
给你一个 m x n 的矩阵 matrix 。如果这个矩阵是托普利茨矩阵,返回 true ;否则,返回 __false _。_
如果矩阵上每一条由左上到右下的对角线上的元素都相同,那么这个矩阵是 __托普利茨矩阵 。
示例 1:

输入: matrix = [[1,2,3,4],[5,1,2,3],[9,5,1,2]]
输出: true
解释:
在上述矩阵中, 其对角线为:
"[9]", "[5, 5]", "[1, 1, 1]", "[2, 2, 2]", "[3, 3]", "[4]"。
各条对角线上的所有元素均相同, 因此答案是 True 。
示例 2:

输入: matrix = [[1,2],[2,2]]
输出: false
解释:
对角线 "[1, 2]" 上的元素不同。
提示:
m == matrix.lengthn == matrix[i].length1 <= m, n <= 200 <= matrix[i][j] <= 99
进阶:
- 如果矩阵存储在磁盘上,并且内存有限,以至于一次最多只能将矩阵的一行加载到内存中,该怎么办?
- 如果矩阵太大,以至于一次只能将不完整的一行加载到内存中,该怎么办?
解题思路
一个矩阵是 托普利茨矩阵 的条件是:任意从左上到右下的对角线上的所有元素都相等。 换句话说,对于矩阵的任意位置 (i, j),如果其右下方的元素 (i+1, j+1) 存在,则 matrix[i][j] == matrix[i+1][j+1]。
基础版
遍历矩阵:
- 从第一行和第一列开始,遍历所有可能的对角线上的元素。
- 检查是否满足
matrix[i][j] == matrix[i+1][j+1]。
注意边界条件:
- 如果是矩阵的最后一行或者最后一列,则无需再进行比较。
复杂度分析
- 时间复杂度:
O(m * n),遍历矩阵中除最后一行和最后一列的所有元素。 - 空间复杂度:
O(1),原地比较,不使用额外空间。
进阶版
行内存加载限制:
- 由于内存限制,一次只能加载矩阵的一行。可以采用以下策略:
- 逐行加载矩阵,保存上一行的内容到一个数组中。
- 加载当前行时,比较当前行与上一行相邻的元素是否相等。
- 只需存储两行的内容,空间复杂度为
O(n)。
- 由于内存限制,一次只能加载矩阵的一行。可以采用以下策略:
不完整行内存限制:
- 假设一次只能加载矩阵的一部分,可以使用滑动窗口方法。
- 对于每次加载的一部分行或列,只记录必要的对角线元素,逐步验证其一致性。
- 例如,可以用一个固定长度的数组存储对角线的首元素,逐步更新和比较。
- 假设一次只能加载矩阵的一部分,可以使用滑动窗口方法。
复杂度分析
- 时间复杂度:
O(m * n),遍历矩阵中除最后一行和最后一列的所有元素。 - 空间复杂度:
O(n),只需存储一行的内容。
代码
/**
* @param {number[][]} matrix
* @return {boolean}
*/
var isToeplitzMatrix = function (matrix) {
const m = matrix.length;
const n = matrix[0].length;
for (let i = 0; i < m - 1; i++) {
for (let j = 0; j < n - 1; j++) {
if (matrix[i][j] !== matrix[i + 1][j + 1]) {
return false;
}
}
}
return true;
};
/**
* @param {number[][]} matrix
* @return {boolean}
*/
var isToeplitzMatrix = function (matrix) {
let prevRow = matrix[0];
for (let i = 1; i < matrix.length; i++) {
for (let j = 1; j < matrix[i].length; j++) {
if (matrix[i][j] !== prevRow[j - 1]) {
return false;
}
}
prevRow = matrix[i];
}
return true;
};
相关题目
| 题号 | 标题 | 题解 | 标签 | 难度 | 力扣 |
|---|---|---|---|---|---|
| 422 | 有效的单词方块 🔒 | 数组 矩阵 | 🟢 | 🀄️ 🔗 |
